
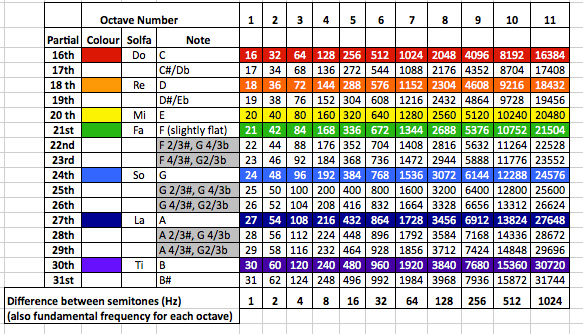
16-tone tuning is a system where all note frequencies are whole number. It is related to just intonation and scientific tuning. It is also built upon the overtones of a vibrating string. By understanding the mathematics behind musical intervals, this may aid in learning music, and also pave the way for the development of new music.
The lowest octave of this 16-tone tuning system starts at 16 Hz (the 16th partial of 1 Hz) and extends to 31 Hz (31st partial). For each ascending octave, the pitches of the previous 16 tones are doubled (i.e. the second octave spans from 32–62 Hz, the third from 64–124, and so on).
Assigning exact numerical relationships to tones and their intervals can make responding to notes a simpler process. Visual processing can be calibrated to frequency without the need to account for decimal places. Because the consonant tones found in this system are not considered “slightly out of tune” (as with equal temperament), it may be more likely for relative harmonics to be visually represented as having a tangible connection to a fundamental frequency.
Consonance and dissonance can then be viewed as more of a spectrum, as opposed to extremes. Certain intervals are close to being perfectly consonant, but by using rational numbers we can quantify consonance and dissonance precisely. For example, the interval F-C in equal temperament is a “perfect fifth”. Using the 16-tone tuning method, the lowest F on the instrument can be labelled “21” (its value in Hertz) and the C above it can be labelled “32”, i.e. a relation of 32/21, or 1.5238. This is an interval that is slightly larger than a perfect fifth, which may serve a harmonic function in the future.